The adjusted expectations are approximately
These quantities, which are constructed
solely from belief specifications, are functions of the (unobserved)
data; informally they are akin to estimators in the traditional
sense.
Generally speaking, it is difficult
to tell whether a small coefficient truly indicates unimportance because
of the different expectations and scalings of 
and 
.
For this reason, we also examine the standardised adjusted expectations,
being approximately
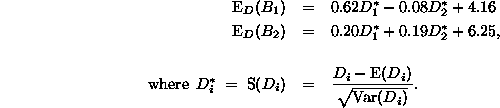
is our general notation for the standardised representation of a
quantity. Now each coefficient multiplies a quantity that has
expectation zero and variance unity, so that the coefficients are more
readily comparable. (The constants added in each case are the initial
expectations for 
and 
.) We see that the adjusted
expectation for depends essentially on , plus a base
value of 4.16; whereas the adjusted expectation for depends
upon a rather larger base value, plus essentially an average of the
before-and-after blood-glucose readings.