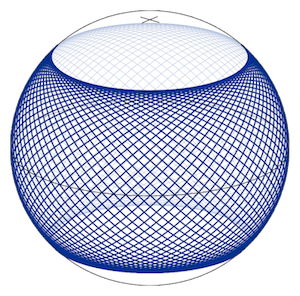

DescriptionIt is well known that the shortest path between two points in Euclidean space is a straight line. Everybody, even if they are not at all interested in mathematics, uses this fact in every day life when they walk or drive from point A to point B. The generalization of straight lines on a curved surface (or more generally a higher dimensional Riemannian manifold) leads to the notion of a geodesic. A geodesic is a curve which, at least for nearby points, minimizes the distance within the surface or manifold. The physical motivation for a geodesic is a curve whose acceleration vector is orthogonal to the space. This approach leads to the explicit description of geodesics as solutions of a specific second order non-linear differential equation. E.g., on a sphere with the standard rotationally invariant metric, all the geodesics are parts of closed great circles.In this project we will investigate on particular results about geodesics. On a surface of revolution in Euclidean 3-space with rotation axis g, geodesics preserve a particular expression in the distance to the axis g and the angle against the parallels. This fact is known as Clairaut's Theorem. A more fancy way of putting it is that the geodesic flow on surfaces of revolution is integrable. There is another well-known example of a surface with an integrable geodesic flow: the ellipsoid. The ellipsoid is given by the equation
If all three parameters a,b,c > 0 are different then the ellipsoid is not a surface of revolution and has precisely four umbilic points, and every geodesic starting at an umbilic point will pass through another umbilic point and so on. Moreover, we have the following limiting result: Keeping a and b fixed and letting c tend to zero, the geodesics on the ellipsoid converge to the trajectories of a plane elliptic billiard. Another stunning result is the fact that whatever metric one chooses on a topological two-dimensional sphere there is always at least one closed geodesic. This is clear for the rotationally invariant metric but not at all obvious, if we start to disturb the metric. The proof uses both geometric and topological tools. In fact, a much stronger result is true which was obtained in 1991 by John Franks (USA) and Victor Bangert (Germany), combining their mutually disjoint methods: for any metric on a two-sphere there are infinitely many closed geodesics. This highly celebrated stronger result, however, is far beyond the scope of this project. There are many other topics related to geodesics on surfaces and manifolds. This means that the project is open ended and can lead to various directions of investigations different from the examples mentioned above. For example there is the potential to study geodesics in more general non-smooth geometries like Alexandrov spaces and also the possibility to work with Maple to produce visualizations of geodesics on surfaces. Prerequisites and CorequisitesDifferential Geometry III is essential and Riemannian Geometry IV is advisable.Some ResourcesArthur L. Besse Manifolds all of whose geodesics are closedManfredo P. do Carmo Differential geometry of curves and surfaces Jürgen Jost Riemannian geometry and geometric analysis John W. Milnor Topology from the differentiable viewpoint Michael Spivak A comprehensive introduction to differential geometry, Volume III
|
email: W Klingenberg